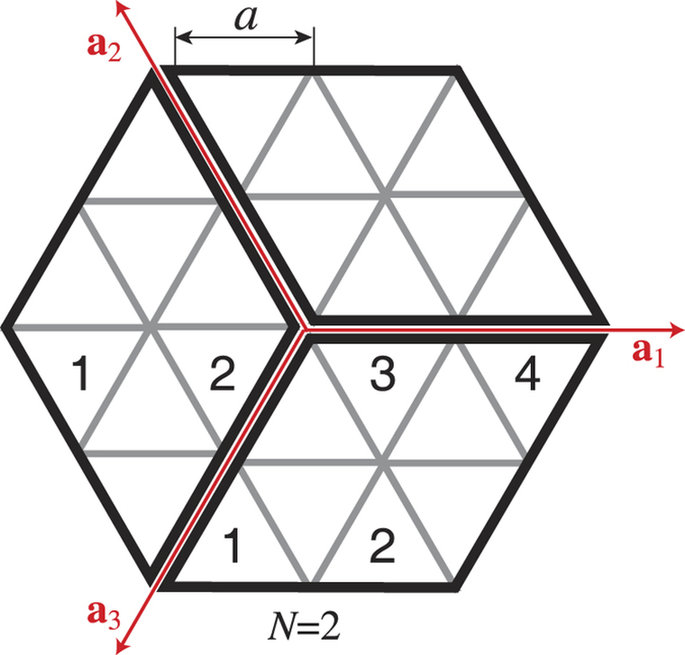
In a hexagon, there are 9 distinct parallelograms that can be formed by selecting sets of 4 vertices. These include 3 inner diagonals, 3 outer diagonals, and 3 cases involving one pair of opposite sides and two pairs of adjacent sides. This count doesn’t consider rotations or reflections as separate parallelograms.
What Are The Basic Concepts?
A hexagon is a polygon with six sides and six angles. It is a two-dimensional shape that has six straight sides, and all of its internal angles sum up to 720 degrees.
Additionally, hexagons come in various forms, such as regular hexagons (all sides and angles are equal) and irregular hexagons (sides and angles may have different lengths and measures).
Characteristics of a Parallelogram:
A parallelogram is a quadrilateral with opposite sides that are parallel and equal in length. It has four angles, with opposite angles being equal. Parallelograms also possess other essential properties:
Opposite sides are congruent (equal in length).
- Opposite angles are congruent.
- Consecutive angles are supplementary (they add up to 180 degrees).
- Diagonals bisect each other (they intersect at their midpoints).
How Can You Identify Parallelograms in a Hexagon?
Explanation of Different Types of Parallelograms in a Hexagon:
- Inner Parallelograms: These are parallelograms that can be found entirely within the interior of the hexagon.
Moreover, they are formed by connecting two pairs of non-adjacent vertices of the hexagon. These parallelograms can have varying orientations and sizes..
- Edge-Based Parallelograms: These are parallelograms that share one or more sides with the hexagon. In addition, they can be formed by selecting two adjacent sides of the hexagon and then extending the lines parallel to the opposite sides of the hexagon.
How Can You Count Parallelograms in a Hexagon?
To count the parallelograms in a hexagon, we need to systematically analyze and categorize them based on their positions and orientations within the hexagon. Moreover, this involves breaking down the counting process into distinct steps to ensure accuracy.
Breakdown of Counting Process for Clarity:
Counting Inner Parallelograms:
- Identify potential pairs of non-adjacent vertices in the hexagon.
- Draw lines connecting these pairs to form potential inner parallelograms.
- Verify if these lines are parallel and of equal length to form a valid parallelogram.
- Count all the valid inner parallelograms.
Counting Edge-Based Parallelograms:
- Select a side of the hexagon.
- Extend lines parallel to this side from the other side(s) adjacent to it.
- Identify the points where these lines intersect the adjacent sides.
- Verify if the formed figure is a parallelogram by checking side lengths and angles.
- Repeat this process for all sides of the hexagon.
- Count all the valid edge-based parallelograms.
What Are The Examples Of That?
Visual Examples of Counting Parallelograms in a Hexagon:
Diagrams Illustrating the Counting Process: Visual representations of hexagons with labeled vertices and lines connecting them can help illustrate the process of identifying and counting different parallelograms.
Moreover, these diagrams demonstrate how inner and edge-based parallelograms are formed and labeled.
Step-by-Step Examples: Provide step-by-step walkthroughs of specific hexagonal shapes, showing how to identify and count the different types of parallelograms present.
In addition, break down each example by detailing the selection of vertices, drawing lines, and confirming the parallelogram criteria.
What Are The General Formula (if Applicable) ?
If there’s a pattern that emerges from the process of counting parallelograms in hexagons, you can introduce a generalized formula. This formula could be based on the number of vertices and sides in the hexagon.
Explanation of Variables and Their Significance:
If you establish a formula, explain each variable’s significance. For instance, if ‘n’ represents the number of vertices, ‘m’ denotes the number of sides, and ‘p’ symbolizes the number of parallelograms.
Moreover it clarifies the relationships between them. Highlight how this formula simplifies the counting process for larger hexagons.
What Are The Real-world Applications?
Mention of Situations Where Knowledge of Counting Parallelograms Is Useful:
- Architectural and Design Planning: When designing patterns or structures with hexagonal elements, knowing how to count parallelograms can aid in creating aesthetically pleasing arrangements.
- Manufacturing and Fabrication: Moreover, Hexagonal patterns are used in various manufacturing processes. Understanding parallelogram counts can optimize material usage and design efficiency.
Problem-solving Scenarios Involving Hexagonal Patterns:
- Tiling and Flooring: When tiling a floor with hexagonal tiles, you can optimize the layout by considering parallelogram arrangements.
- Puzzle and Game Design: Creating puzzles or games involving hexagons often requires counting parallelograms to determine solutions or strategies.
What Are The Challenges and Considerations?
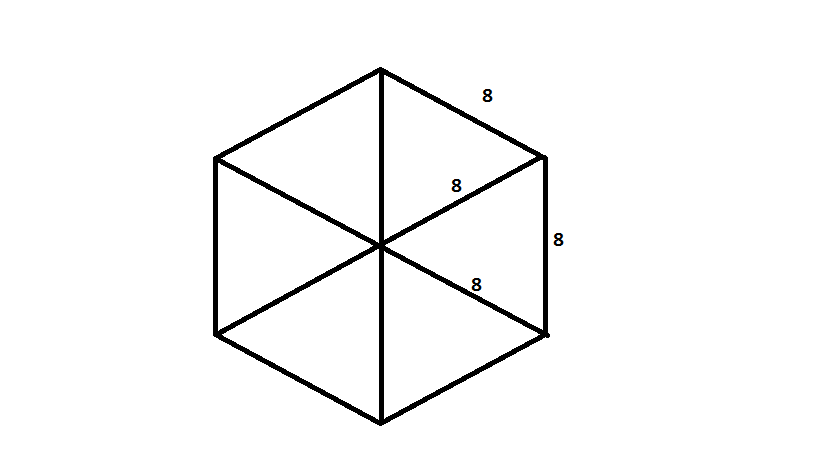
Potential Challenges in Accurately Counting Parallelograms:
- Overlapping Parallelograms: Parallelograms can overlap within a hexagon, leading to potential double-counting.
- Complex Arrangements: In intricate hexagonal patterns, distinguishing between parallelograms can be challenging.
Tips for Overcoming Common Counting Errors:
- Systematic Approach: Use a systematic method, such as starting with inner parallelograms and then considering edge-based ones.
- Visual Verification: Double-check your counts with visual aids and diagrams.
- Practice and Patience: Practice counting parallelograms in simpler shapes to improve accuracy.
FAQs
What are inner parallelograms in a hexagon?
Inner parallelograms are those that are fully enclosed within the interior of the hexagon. They are formed by connecting two pairs of non-adjacent vertices of the hexagon.
How do you identify edge-based parallelograms in a hexagon?
Edge-based parallelograms share one or more sides with the hexagon. They can be formed by selecting two adjacent sides of the hexagon and extending lines parallel to the opposite sides.
Is there a systematic way to count parallelograms in a hexagon?
Yes, a systematic approach involves first counting inner parallelograms by connecting non-adjacent vertices and then counting edge-based parallelograms by extending lines from adjacent sides.
What challenges can arise when counting parallelograms in a hexagon?
Challenges include the possibility of overlapping parallelograms leading to overcounts, and in complex hexagonal patterns, distinguishing between different parallelograms can be tricky.
How can I avoid errors when counting parallelograms in a hexagon?
To avoid errors, use a systematic approach, visually verify counts with diagrams, and practice with simpler shapes. Take your time and be meticulous in your analysis.
Why is understanding the count of parallelograms in a hexagon important?
Understanding this count not only enhances geometric knowledge but also develops problem-solving skills, critical thinking, and the ability to analyze complex patterns in various applications.
Conclusion:
In conclusion, understanding the intricacies of counting parallelograms within a hexagon goes beyond a mere mathematical exercise.
Moreover, this exploration provides valuable insights into the geometric properties of hexagons and parallelograms, as well as their practical applications in various fields. Through the detailed examination of the topic, several key takeaways emerge:
Geometric Insight: The study of parallelograms within hexagons enhances our grasp of geometric relationships, angles, sides, and patterns. However, it fosters a deeper appreciation for the symmetrical and proportional nature of these shapes.
Systematic Analysis: The methodical approach to counting parallelograms not only ensures accuracy but also sharpens analytical skills. Moreover, the systematic breakdown of the counting process equips us with a structured problem-solving mindset.
Generalization: The possibility of deriving a formula for parallelogram counting in hexagons showcases the power of abstraction and generalization in mathematics. Such a formula, if applicable, can streamline complex counting tasks.
Real-world Relevance: The knowledge gained from counting parallelograms finds practical applications in architecture, design, manufacturing, puzzles, and games. It empowers us to optimize layouts, utilize resources efficiently, and devise creative solutions.
Challenge and Resilience: The challenges inherent in accurately counting parallelograms underscore the importance of attention to detail and perseverance. Overcoming these challenges hones our problem-solving abilities and resilience.